NACA 4 digit airfoil generator (NACA 2412 AIRFOIL)

NACA 2412 Airfoil M=2.0% P=40.0% T=12.0%
  1.000084  0.001257
  0.998557  0.001575
  0.993984  0.002524
  0.986392  0.004086
  0.975825  0.006231
  0.962343  0.008922
  0.946027  0.012110
  0.926971  0.015740
  0.905287  0.019752
  0.881104  0.024079
  0.854565  0.028653
  0.825830  0.033404
  0.795069  0.038260
  0.762469  0.043149
  0.728228  0.048000
  0.692554  0.052741
  0.655665  0.057302
  0.617788  0.061615
  0.579155  0.065609
  0.540008  0.069220
  0.500588  0.072381
  0.461143  0.075034
  0.421921  0.077122
  0.383032  0.078574
  0.344680  0.079198
  0.307289  0.078941
  0.271106  0.077802
  0.236371  0.075794
  0.203313  0.072947
  0.172151  0.069309
  0.143088  0.064941
  0.116313  0.059918
  0.091996  0.054325
  0.070289  0.048257
  0.051324  0.041808
  0.035214  0.035076
  0.022051  0.028152
  0.011907  0.021120
  0.004833  0.014049
  0.000860  0.006997
  0.000000  0.000000
  0.002223 -0.006689
  0.007479 -0.012828
  0.015723 -0.018404
  0.026892 -0.023408
  0.040906 -0.027826
  0.057669 -0.031651
  0.077071 -0.034878
  0.098987 -0.037507
  0.123281 -0.039546
  0.149805 -0.041013
  0.178401 -0.041934
  0.208902 -0.042346
  0.241131 -0.042294
  0.274904 -0.041834
  0.310028 -0.041027
  0.346303 -0.039941
  0.383522 -0.038644
  0.421644 -0.037174
  0.460397 -0.035444
  0.499412 -0.033493
  0.538451 -0.031373
  0.577279 -0.029138
  0.615658 -0.026833
  0.653352 -0.024500
  0.690129 -0.022172
  0.725762 -0.019880
  0.760029 -0.017649
  0.792716 -0.015499
  0.823619 -0.013448
  0.852541 -0.011510
  0.879302 -0.009701
  0.903730 -0.008033
  0.925669 -0.006520
  0.944979 -0.005174
  0.961536 -0.004008
  0.975232 -0.003035
  0.985978 -0.002265
  0.993705 -0.001708
  0.998361 -0.001370
  0.999916 -0.001257

NACA 4 digit airfoil specification

• M is the maximum camber divided by 100. In the example M=2 so the camber is 0.02 or 2% of the chord
• P is the position of the maximum camber divided by 10. In the example P=4 so the maximum camber is at 0.4 or 40% of the chord.
• XX is the thickness divided by 100. In the example XX=12 so the thiickness is 0.12 or 12% of the chord.

NACA 4 digit airfoil calculation

The NACA airfoil section is created from a camber line and a thickness distribution plotted perpendicular to the camber line.

The equation for the camber line is split into sections either side of the point of maximum camber position (P). In order to calculate the position of the final airfoil envelope later the gradient of the camber line is also required. The equations are:

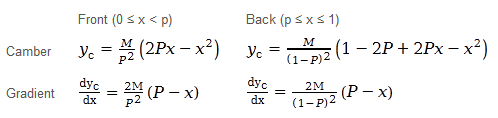
The thickness distribution is given by the equation:

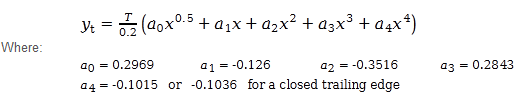
• The constants a0 to a4 are for a 20% thick airfoil. The expression T/0.2 adjusts the constants to the required thickness.
• At the trailing edge (x=1) there is a finite thickness of 0.0021 chord width for a 20% airfoil. If a closed trailing edge is required the value of a4 can be adjusted.
• The value of yt is a half thickness and needs to be applied both sides of the camber line.

Using the equations above, for a given value of x it is possible to calculate the camber line position Yc, the gradient of the camber line and the thickness. The position of the upper and lower surface can then be calculated perpendicular to the camber line.

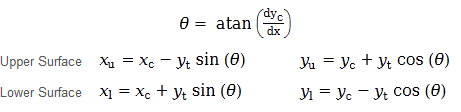
The most obvious way to to plot the airfoil is to iterate through equally spaced values of x calclating the upper and lower surface coordinates. While this works, the points are more widely spaced around the leading edge where the curvature is greatest and flat sections can be seen on the plots. To group the points at the ends of the airfoil sections a cosine spacing is used with uniform increments of β

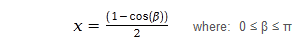
References

• Geometry for Aerodynamicists
• Computer Program To Obtain Ordinates for NACA Airfoils
• The NACA Airfoil Series